by Michael C. Sullivan
Northwestern University
August 17 1994
Published in the March 1995 issue of "Undergraduate Mathematics Education Trends".
There is much I like about the calculus reform movement. Too often calculus and precollege mathematics' students were made to memorize bulky formulas and arcane tricks. There was little understanding on the intuitive or theoretical levels.
I have in my few years of teaching tried to avoid the rigidities of traditional approaches. Being a visual thinker, I would integrate graphical/intuitive perspectives with more formal arguments. Having worked in digital signal processing I even incorporated numerical ideas where I thought appropriate.
Naturally when I first heard of reform efforts and the "rule of three" (to integrate graphical, numerical and analytical views of a given topic) I was excited. However, it seems I wrongly understood analytical to include theory and proofs. According to Martin Flashman (UME Trends, July 94) in the "new wave" of calculus textbooks, "[t]heorems that traditionally provided the foundations have been removed or downplayed when less formal justifications seem convincing." While attending the first Project NExT Conference, a speaker on the Harvard Curriculum made it clear that there were to be no proofs. When I asked why if we wanted to enrich our students intellectual development, theory was being reduced, he replied, after some prodding: "Most of our students are going to be grunt engineers."
Now I understood. We are educating Dilbert. The engineer of the past sat in his office and looked up things in tables. The modern engineer, sits at his or her cubicle and stares at graphs on a computer. In the past calculus texts paid lip service to theory, now all pretense is to be dropped.
Why study proofs? Because the concept of the mathematical proof is a major achievement of the human intellect. It may not be up there with fire or language, but it surely out ranks the graphics calculator, and I believe even the computer. Any educated person should have some exposure to the mathematical proof just as everyone should know something of art and astronomy. Education, including math education, is not just a function of vocational utility. Indeed, ultimately, education should empower and enlighten people so that they will not submit to stifling life styles and work conditions.
How much weight should proofs be given in first year calculus? My belief is 10% or there abouts. That is 10% of students' final grade should be based on their ability to prove mathematical statements. A test that has 10 questions should include one proof, hopefully an original one.
Using this ``10% rule'' calculus would be both a pump and a filter. Students who don't like the proofs, but still do well, know that they should major in an applied field. Those who get high A's or who just decide proofs are cool, can be encouraged to try some ``pure math'' courses. They may wind up doing math research some day or just come way feeling they have gained a deeper insight into nature. Either case is fine with me.
Now I am not claiming that traditional calculus texts or teaching methods have been doing a good job in dealing with theory. Much of my criticism of the reform movement holds for traditional approaches. While there have always been teachers who could hammer the concepts home, the challenge for the reformers is to find effective and portable ways to teach theory, not to bury it under the gloss. Allow me to give two examples.
Example 1: Most calculus texts give no motivation for the theorem:
In the spirit of the
reform movement I believe one should start with a graphical
illustration that 
looks like 
.
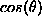Hence we have a conjecture. Since 
is just a
quarter cycle translate of 
, if our conjecture
holds we have a corollary, 
is a solution of
(Or 
has an orbit of period four.)
With their marvelous calculators, students could numerically
see that 
looks a lot like 
.
But how to be sure? In math as in life, what you see is not
always what you get.
The proof should be done in full detail.
Of course many students will whine. It's a free
country. They can whine if they want. This doesn't negate my
right to teach truth.
Test Problem: Define a "sine-like" function f(x) using semi-circular arcs as in the figure below. Graph y=f'(x). Write a paragraph explaining your answer.
I will wager that most new wave students will draw a "cosine-like" graph while most traditional students would be completely stumped. But maybe when the tests come back, they will see why that proof was needed. (Question: What happens if, instead of graphing the slope of the tangent line, you graph its angle of inclination?)
Example 2: Find a formula for
With some effort students might become convinced that 
grows
like 
. Maybe it's a quadratic. Using n=1, 2, and 3 we get
three linear equations in three unknowns. Solving gives 
. More numbers can be tested. But how
to
prove it? There is a nice pictorial "proof" that should be used.
Now try 
, 
, etc. Pictorial proofs start
to get rather tricky. Now is the time to introduce proof by
induction. And what would be so wrong if one test question asked for
an induction proof? Now not everyone will get it. But then not
everyone gets an A. Math, like life, can be tough. But we must not teach
down to only the lowest common denominator.
* Dilbert is a comic strip by Scott Adams about an engineer named Dilbert. It is distributed by United Feature Syndicate, Inc. "Dilbert" is a registed trademark.